Foundations of Financial Management: An Analysis (part 47)

“Develop success from failures. Discouragement and failure are two of the surest stepping stones to success.” 

– Dale Carnegie

The Capital Budgeting Decision (part C)

by

Charles Lamson

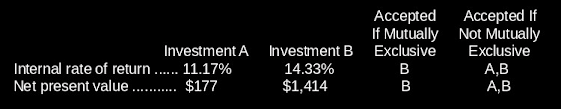
Selection Strategy

In both the internal rate of return and net present value methods covered in last post, the profitability must equal or exceed the cost of capital for the project to be potentially acceptable. However, other distinctions are necessary---namely, whether the projects are mutually exclusive or not. If investments are mutually exclusive, the selection of one alternative will preclude the selection of any other alternative. Assume we are going to build a specialized assembly plant, and four major international cities are under construction, only one of which will be picked. In this situation we select the alternative with the highest acceptable yield or the highest net present value and disregard all others. Even if certain locations provide a marginal return in excess of the cost of capital, assumed to be 10 percent, they will be rejected. In the table below, the possible alternatives are presented.

Among the mutually exclusive alternatives, only Bangkok would be selected. If the alternatives were not mutually exclusive (for example, much-needed multiple retail outlets), we would accept all of the alternatives that provide a return in excess of our cost of capital, and only Singapore would be rejected.

Applying this logic to Investments A and B in the prior post and assuming a cost of capital of 10 percent, only Investment B would be accepted if the alternatives were mutually exclusive, while both would clearly qualify if they were not mutually exclusive.

The discussion to this point has assumed the internal rate of return and net present value methods will call for the same decision. Although this is generally true, there are exceptions. Two rules may be stated:

1. Both methods will accept or reject the same investments based on minimum return or cost of capital criteria. If an investment has a positive net present value, it will also have an internal rate of return in excess of the cost of capital.

2.  In certain limited cases, however, the two methods may give different answers in selecting the best investment from a range of acceptable alternatives.

Reinvestment Assumption

It is only under this second state of events that a preference for one method over the other must be established. A prime characteristic of the internal rate of return is the reinvestment assumption that all inflows can be reinvested at the yield from a given investment. For example, in the case of the aforementioned Investment A yielding 11.17 percent, the assumption is made that the dollar amounts coming in each year can be reinvested at that rate. For Investment B, with a 14.33% internal rate of return, the new funds are assumed to be reinvested at this high rate. The relationships are presented in Table 5.

Table 5 The reinvestment assumption---internal rate of return ($10,000) investment)

For Investments with a very high IRR, it may be unrealistic to assume that reinvestment can occur at an equally high rate. The net present value, depicted in Table 6, makes the more conservative assumption that each inflow can be reinvested at the cost of capital or discount rate. 

Table 6 The reinvestment assumption---net present value ($10,000 investment)

The reinvestment of assumption under the net present value method allows for a certain consistency. Inflows from each project are assumed to have the same (though conservative) investment opportunity. Although this may not be an accurate picture for all firms, net present value is generally the preferred method.

Modified Internal Rate of Return You should also be aware that there is a recently developed methodology that combines the reinvestment assumption of the net present value method (cost of capital) with the internal rate of return. This process is termed the modified internal rate of return (MIRR). The analyst searches for the discount rate that will equate the future value of the inflows, each growing at the cost of capital, with the investment.

In terms of a formula, we show:

The terminal value of the inflows is equal to the sum of the future value of each inflow reinvested at the cost of capital. MIRR is the modified internal rate of return discount rate that equates the terminal (final) value of the inflows with the investment. As an example, assume $10,000 will produce the following inflows for the next three years:

The cost of capital is 10%.

First, determine the terminal value of the inflows at a growth rate equal to the cost of capital. The assumption is that the inflows will come at the end of each period.

To determine the modified internal rate of return, we calculate the yield on the investment. The formula to help determine yield is Formula 2. PV is the investment value and FV is the terminal value of the inflows.

We go to Table 2 from last post for three periods and a tabular value of .641. We see the yield or modified internal rate of return is 16 percent. Had we computed the conventional internal rate of return used throughout these posts dealing with the capital budgeting decision, the answer would have been approximately 21 percent, which is based on reinvestment at the internal rate of return.

The modified internal rate of return, using the more realistic assumption of reinvestment at the cost of capital, gives a more conservative, perhaps better, answer. For that reason, you should be familiar with it. However and the balance of these posts covering the capital budgeting decision, when the internal rate of return is called for, we will use the traditional internal rate of return rather than the modified internal rate of return because of the former's wider usage. 

*MAIN SOURCE: BLOCK & HIRT, 2005, FOUNDATIONS OF FINANCIAL MANAGEMENT, 11TH ED., PP. 358-361*

end

In the wake of the clippers ships, on board the Royal Clipper (Documenta...

Foundations of Financial Management: An Analysis (part 46)

“Money cannot buy peace of mind. It cannot heal ruptured relationships, or build meaning into a life that has none.” 

– Richard M. DeVos

The Capital Budgeting Decision (Part B)

by

Charles Lamson

Methods of Ranking Investment Proposals

Three widely used methods for evaluating Capital expenditures will be considered, along with the shortcomings and advantages of each. 

1. Payback method.

2.  internal rate of return.

3.  Net Present Value.

The first method, while not conceptually sound, is often used. Approaches 2 and 3 are more acceptable, and one or the other should be applied to most situations.

Payback Method

Under the payback method, we compute the time required to recoup the original investment. Assume we are called on to select between investments A&B in Table 3.

Table 3 Investment alternatives

The payback period for Investment A is two years, while Investment B requires 3.8 years. In the latter case, we recovered $6,000 in the first three years, leaving us with a need for another $4,000 to recoup the full $10,000 investment. Since the fourth year has a total inflow of $5,000, $4,000 represents 0.8 of that value. Thus the payback period for Investment B is 3.8 years.

In using the payback method to select Investment A, two important considerations are ignored. First there is no consideration of inflows after the cutoff period. The $2,000 in year 3 for Investment A in Table 3 is ignored, as is the $5,000 in year 5 for Investment B. Even if the $5,000 were $50,000, it would have no impact on the decision under the payback method.

Second, the method fails to consider the concept of the time value of money. If we had two $10,000 investments with the following inflow patterns, the payback method would rank them equally. 

Although both investments have a payback period of two years, the first alternative is clearly superior because the $9,000 comes in the first year rather than the second.

The payback method does have some features that help to explain its use by U.S. corporations. It is easy to understand, and it emphasizes liquidity. An investment must recoup the initial investment quickly or it will not qualify (most corporations use a maximum time horizon of three to five years). A rapid payback may be particularly important to firms in industries characterized by rapid technological developments.

Nevertheless the payback method, concentrating as it does on only the initial use of investment, fails to discern the optimum or most economic solution to a capital budgeting problem. The analyst is therefore required to consider more theoretically correct methods.

Internal Rate of Return

The internal rate of return (IRR) calls for determining the yield on an investment, that is, calculating the interest rate that equates the cash outflows (cost) of an investment with the subsequent cash flows. The simplest case would be an investment of $100 that provides $120 after 1 year, or a 20 percent internal rate of return. For more complicated situations, we use Table 2 from part 31 of this analysis (present value of a single amount) and Table 1 from part 31 (present value of an annuity, and the techniques described in parts 26-38 of this analysis dealing with the time value of money and valuation and rates of return. For example, a $1,000 investment returning an annuity of $244 per year for five years provides an internal rate of return of 7 percent, as indicated by the following calculations.

The solution at 10% is $177 away from $10,000. Actually the solution is ($177/$303) percent of the way between 10 and 12 percent.Since there is a two percentage-point difference between the two rates used to evaluate the cash inflows, we need to multiply the fraction by 2% and then add our answer to 10% for the final answer of:

10% + ($177/$303)(2%) = 11.17% IRR

In Investment B the same process will yield an answer of 14.33%.

The use of the internal rate of return calls for the prudent selection of Investment B in preference to Investment A, the exact opposite of the conclusion reached under the payback method.

The final selection of any project under the internal rate of return method will also depend on the yield exceeding some minimum cost standard, such as the cost of capital to the firm.

Net Present Value

The final method of investment selection is to determine the net present value of an investment. This is done by discounting back the inflows over the life of the investment to determine whether they equal or exceed the required investment. The basic discount rate is usually the cost of capital to the firm. Thus inflows that arrive in later years must provide a return that at least equals the cost of financing those returns. If we once again evaluate Investments A and B using an assumed cost of capital, or a discount rate, of 10 percent---we arrive at the following figures for net present value. 

While both proposals appear to be acceptable, Investment B has a considerably higher net present value than Investment A. Under most circumstances the net present value and internal rate of return methods give theoretically correct answers, and the subsequent discussion will be restricted to these two approaches. A summary of the various conclusions reached under the three methods is presented in Table 4.

Table 4 Capital budgeting results

*MAIN SOURCE: BLOCK & HIRT, 2005, FOUNDATIONS OF FINANCIAL MANAGEMENT, 11TH ED., PP. 354-358*

end

Foundations of Financial Management: An Analysis (part 45)

“The habit of saving is itself an education; it fosters every virtue, teaches self-denial, cultivates the sense of order, trains to forethought, and so broadens the mind.” 

– T.T. Munger

The Capital Budgeting Decision

by

Charles Lamson

The decision on capital outlays is among the most significant a firm has to make. A decision to build a new plant or expand into a foreign market may influence the performance of the firm over the next decade.

The capital budgeting decision involves the planning of expenditures for a project with a life of at least one year, and usually considerably longer. In the public utilities sector, the time horizon of 25 years is not unusual. The capital expenditure decision requires extensive planning to ensure that engineering and marketing information is available, product design is complemented, necessary patterns are acquired, and the capital markets are tapped for the necessary funds. Throughout the next few posts we will use techniques developed under the discussion of the time value of money to equate future cash flows to the present, while using the cost of capital as the basic discount rate.

It should be pointed out that capital budgeting is not only important to people in finance or accounting, it is essential to people throughout the business organization. For example, a marketing or production manager who is proposing a new product must be familiar with the capital budgeting procedures of the firm. If he or she is not familiar with the concepts presented in these next several posts, the best idea in the world may not be approved because it has not been properly evaluated and presented. You must not only be familiar with your product, but also with its financial viability.

In the next few posts capital budgeting is studied under the following major topical headings: administrative considerations, accounting flows versus cash flows, methods of ranking investment proposals, selection strategy, capital rationing, combining cash flow analysis and selection strategy, and the replacement decision. Later in the chapter, taxes and their impact on depreciation and capital budgeting decisions are emphasized.

Administrative Considerations

A good capital budgeting program requires that a number of steps be taken in the decision-making process.

1. Search for and discovery of investment opportunities.

2.  Collection of data.

3.  Evaluation and decision making.

4.  Reevaluation and adjustment.

The search for new opportunities is often the least emphasized, though perhaps the most important, of the four steps. The collection of data should go beyond engineering data and market surveys and should attempt to capture the relative likelihood of the occurrence of various events. The probabilities of increases or slumps in product demand may be evaluated from statistical analysis, while other outcomes may be estimated subjectively.

After all data have been collected and evaluated, the final decision must be made. Generally determinations involving relatively small amounts of money will be made at the department or division level, while major expenditures can be approved only by top management. A constant monitoring of the results of a given decision may indicate that a new set of probabilities must be developed, based on first year experience, and the initial decision to choose Product A over product B must be reevaluated and perhaps reversed. The preceding factors are Illustrated in Figure 1 below.

Accounting Flows versus Cash Flows

In most capital budgeting decisions the emphasis is on cash flow rather than reported income. Let us consider the logic of using cash flow in the capital budgeting process. Because depreciation does not represent an actual expenditure of funds in arriving at profit, it is added back to profit to determine the amount of cash flow generated. Assume the Lamson Corporation has $50,000 of new equipment to be depreciated at $5,000 per year. The firm has $20,000 in earnings before depreciation and taxes and pays 35 percent in taxes. The information is presented in Table 1 to illustrate the key points involved.

Table 1 Cash flow for Lamson Corporation

The firm shows $9,750 in earnings after taxes, but it adds back the noncash deduction of $5,000 in depreciation to arrive at a cash flow figure of $14,750. The logic of adding back depreciation becomes even greater if we consider the impact of $20,000 in depreciation for the Lamson Corporation (Table 2). Net earnings before and after taxes are zero, but the company has $20,000 cash in the bank. 

Table 2 Revised cash flow for Lamson Corporation

To the capital budgeting specialist, the use of cash flow figures is well accepted. However, top management does not always take a similar viewpoint. Assume you are the president of a firm listed on the New York Stock Exchange and must select between two alternatives. Proposal A will provide zero in aftertax earnings and $100,000 in cash flow, while Proposal B, calling for no depreciation, will provide $50,000 in aftertax earnings and cash flow. As president of a publicly traded firm, you have security analysts constantly penciling in their projections of your earnings for the next quarter, and you fear your stock may drop dramatically if earnings are too low by even a small amount. Although Proposal A is superior, you may be more sensitive to aftertax earnings than to cash flow and you may therefore select Proposal B. Perhaps you are overly concerned about the short-term impact of a decision rather than the long-term economic benefits that might accrue.

The financial manager must be sensitive to executives' concessions to short-term pressures. Nevertheless in the material that follows, the emphasis is on the use of proper evaluation techniques to make the best economic choices and assure long-term wealth maximization. 

*MAIN SOURCE: BLOCK & HIRT, 2005, FOUNDATIONS OF FINANCIAL MANAGEMENT, PP.

end

Greenland 1905 - 2005, In the wake of Charcot and the Duke of Orléans on...

On the trail of the Vikings (Documentary, Discovery, History)

Foundations of Financial Management: An Analysis (part 44)

“If you live for having it all, what you have is never enough.” 

– Vicki Robin

Cost of Capital (part F)

by

Charles Lamson

Summary

The cost of capital for the firm is determined by computing the costs of various sources of financing and weighting them in proportion to their representation in the capital structure. The cost of each component in the capital structure is closely associated with the valuation of that source, which we studied in the last several posts. For debt and preferred stock, the cost is directly related to the current yield, with debt adjusted downward it to reflect the tax-deductible nature of interest.

We weigh the elements in the capital structure in accordance with our desire to achieve a minimum overall cost. While that is usually the cheapest form of financing, excessive debt use may increase the financial risk of the firm and drive up the costs of all sources of financing. The wise financial manager attempts to ascertain what debt component will result in the lowest overall cost of capital. Once this has been determined, the weighted average cost of capital is the discount rate we use in present-valuing future flows to ensure we are earning at least the cost of financing.

The marginal cost of capital is also introduced to explain what happens to a company's cost of capital as it tries to finance a large amount of funds. First the company will use up retained earnings, and the cost of financing will rise as higher-cost new common stock is substituted for retained earnings in order to maintain the optimum capital structure with the appropriate debt-to-equity ratio. Larger amounts of financial capital can also cause the individual means of financing to rise by raising interest rates or by depressing the price of the stock because more is sold than the market wants to absorb. 

Review of Formulas 

*MAIN SOURCE: BLOCK & HIRT, 2005, FOUNDATIONS OF FINANCIAL MANAGEMENT, 11TH ED., PP. 331-332*

end